Albert Einstein is famously quoted as saying that, “Compound interest is the eighth wonder of the world. He who understands it earns it. He who doesn’t pays it.” While the quote is unlikely to have actually come from Einstein, the sentiment is quite accurate. Compound interest is a powerful force in the finance world, and understanding how it works is key in your own financial success.
How Simple Interest Works
Before we get into compound interest, it is important to understand how simple interest works. Simple interest is best for situations where money is borrowed for a fixed period of time with one payment to return both principle and interest at once.
For example, imagine your car breaks down and you don’t have enough cash on hand for a down payment. Your rich uncle offers to loan you $2,000 for one year, and you agree to pay 2% interest. At the end of the year, you would have to pay back the $2,000 plus 2% of the $2,000, or $40 in interest. To pay off the loan, you owe your uncle $2,040 even though he lent you only $2,000.
To calculate simple interest, use the simple interest formula below:
Interest = Principal x Interest Rate x Time
To get a better understanding, let’s revisit our example. However, this time your uncle is lending you money at 2% interest per year for 2 years.
Using the simple interest formula, we can calculate the total interest due to your rich uncle.
Interest = $2,000 x 2% x 2 years = $80
As you can see, increasing the number of periods proportionally increases interest due. No matter how many years you borrow the money from your rich uncle, the interest is always going to be $40 per year, or 2% of the original $2,000.
While this is simple to calculate, it is rarely ever used in practice by banks and other financial institutions.
How Compound Interest Works
Banks, credit card issuers, and other financial institutions typically use compound interest. With compound interest, at the end of each time period the accumulated interest is added to the principal before calculating the next period’s interest.
Compound interest is calculated with the formula below:
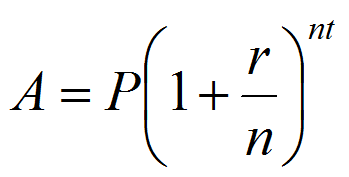
A = Future Value, P = Principal, r = Interest Rate (decimal format), t = Time (in years), n = Number of periods. In real life, there are calculators that handle this for you, so don’t worry about wrapping your head around that. It is much easier to understand with an example.
Continuing with the example above, let’s say your rich uncle agrees to lend you $1,000 for two years at 2% interest, compounded annually. Remember that using simple interest, the total interest cost is $80, so when you pay back your loan, you pay back $2,000 + $80 = $2,080.
Using compound interest, things work a little differently. At the end of the first year, we do the same thing we did at the end of the first year in the simple interest example. $2,000 x .02 = $40. However, because we are compounding, that $40 is added to the principal. At the start of the year instead of owing $2,000 + $40, it is like you had borrowed $2,040.
At the end of the next year, we calculate 2% again, but this time on $2,040. The second year’s interest is $40.80, so you now owe $2,080.80. Sure 80 cents isn’t a lot, but the power of compound interest gets bigger over longer time horizons or when the principal or interest rate is higher.
If your uncle decided to lend you the $2,000 for five years instead of two years, that compounding effect continues. At the end of the third year, you owe $2,122.42. At the end of four years you owe $2,164.86. At the end of the fifth year, you owe $2,208.16. Had we used simple interest, you would have owed only $2,200. The $8.16 difference is due to the power of compounding.
The Cost of Compound Interest: Borrowing
While in our rich uncle scenario above the difference between compound and simple interest was less than $10 over five years, you are more likely to run into compound interest when investing for retirement or borrowing for a mortgage or credit card.
If you were to buy a $250,000 home with 20% down, you would need to borrow $200,000 for a mortgage loan. That is 100 times as big as the example above. Further, you decide on a 30-year mortgage rather than a simple two-year deal like you had with your uncle. Assume current interest rates are around 4%, and that is the rate you locked in for your mortgage. For this example, we will say that all payments are made at the end of the loan rather than monthly. Keep in mind that with actual mortgages, you pay each month so the math looks quite a bit different.
Using simple interest in this situation, you would owe 4% of $200,000 every year for 30 years. That is $8,000 per year for 30 years, or $240,000 in interest over the life of the loan assuming all payments are made at the end of the loan.
Using compound interest for the same loan, you would pay $448,679.50 in interest. That is almost double what you pay with simple interest! Again, remember that mortgage payments are made monthly, so this situation is not exactly what you would run into when borrowing to buy a home.
The Power of Compound Interest: Investing
Compound interest works two ways. While banks and credit card issuers charge interest when you borrow, you can also use compound interest to your advantage when saving and investing.
Savings accounts and dividend paying stock market investments both offer you compound interest and allow you to put your money to work for your own gain.
Let’s say you want to invest in Walmart, which currently pays a 2.88% dividend yield. That means for each $100 of Walmart stock you buy, you will get $2.88 in dividends per year. If you invest $1,000 can simply take these cash dividends and take your $28.80 per year in cash, or you can reinvest in Walmart stock to take advantage of compound returns. $28.80 per year would buy you about 1/3 of a share per year, which helps you slowly build up a bigger stake.
Over enough time or with a big enough investment, that compounding effect can lead to thousands of additional dollars in investment gains or more!
Use Compound Interest to Grow Your Wealth
Knowing how compound interest works helps you better manage your debt and improve your investment savvy. You are empowered to pay off your credit cards or make early payments to lower your debt faster.
At the same time, you now know that increasing your retirement account or other investment contributions can lead to compounding growth. Over time, the impact of compounding interest grows, so invest as much as you can now to take advantage of gains further down the road.
Whatever you do, do not ignore the power of compound interest.
While Einstein may have never actually said a famous quote on compound interest, investor Larry Thurmond said that, “You need to start, you need to be consistent with it, and you need to let compound interest work.”
Tell us about your experience with compound interest below!


The more I read the more convinced I become that every American needs your program. You have done a masterful job of explaining how credit works and how to use credit instead of credit using you! Well done ?